Tables: Exercise-3


Overview:


Questions and Answers Type:MCQ (Multiple Choice Questions).
Main Topic:Data Interpretation.
Data Interpretation Sub-topic:Tables Questions and Answers.
Number of Questions:5 Questions with Solutions.

Directions: Study the following table carefully and answer the questions given below it.


Data related to number of employees in five different organisations in January 2017.


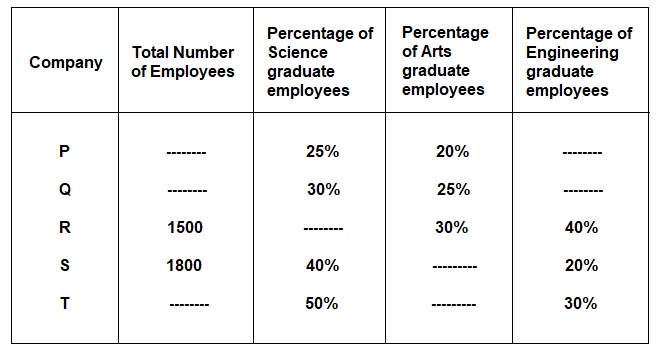
image
  1. The average number of science graduate employees and art graduate employees in company P was 800. What is the total number of employees in company P?

    1. 3556
    2. 3568
    3. 3572
    4. 3576


Answer: (a) 3556

Solution: Total number of science and art graduate employees in company P, $$ = 800 \times 2 = 1600 $$ from the table, $$ 45 \ \% = 1600 $$ then, $$ 100 \ \% = \frac{1600}{45} \times 100 $$ $$ = 3555.56 \approx 3556 $$

  1. Total number of employees in company T was two times the total number of employees in company Q. If the difference between number of art graduate employees in company T and that in company Q was 500, what was the total number of employees in company Q?

    1. 3333
    2. 3432
    3. 3533
    4. 4333


Answer: (a) 3333

Solution: Let total employees in company Q = X

then total employees in company T = 2X

Now thw difference between art graduate employees in company T and that in company Q, $$ \left[(100 - 50 - 30) \ \% \ of \ 2X\right] \\ - \left[25 \ \% \ of \ X\right] = 500 $$ $$ \frac{20 \times 2X}{100} - \frac{25 \times X}{100} = 500 $$ $$ = \frac{40X - 25X}{100} = 500 $$ $$ 15X = 500 $$ $$ X = 3333.34 \approx 3333 $$ Hence, total number of employees in company Q = 3333

  1. If the respective ratio between number of engineering graduate employees and art graduate employees in company S was 5:7, what was the number of engineering graduate employees in company S?

    1. 350
    2. 400
    3. 450
    4. 550


Answer: (c) 450

Solution: Ratio of engineering graduates and art graduates in company S = 5:7

Total number of engineering graduates and art graduates in company , $$ = \left[20 \ \% \ of \ 1800\right] \\ + \left[(100 - 40 - 20) \ \% \ of \ 1800\right] $$ $$ = \frac{20 \times 1800}{100} + \frac{40 \times 1800}{100} $$ $$ = 360 + 720 = 1080 $$ Hence, the number of engineering graduates in company S, $$ = \frac{5}{12} \times 100 $$ $$ = 450 $$

  1. The total number of employees in company R increased by 20% from January 2017 to January 2018. If 50% of the total number of employees in company R in January 2018 were art graduates, what was the number of art graduate employees in company R in January 2018?

    1. 700
    2. 900
    3. 1100
    4. 1200


Answer: (b) 900

Solution: Total number of employees in company R in 2017, $$ = \frac{1500 \times 120}{100} $$ $$ = 1800 $$ Hence, art graduate employees in company R in January 2018, $$ = 50 \ \% \ of \ 1800 $$ $$ = \frac{50 \times 1800}{100} = 900 $$

  1. What was the difference between number of engineering graduate employees and science graduate employees in company R?

    1. 110
    2. 120
    3. 150
    4. 180


Answer: (c) 150

Solution: Number of engineering graduates in company R, $$ = 40 \ \% \ of \ 1500 $$ $$ = \frac{40 \times 1500}{100} = 600 $$ Number of science graduates in company R, $$ = (100 - 30 - 40) \ \% \ of \ 1500 $$ $$ = 30 \ \% \ of \ 1500 $$ $$ = \frac{30 \times 1500}{100} = 450 $$ Hence, Required difference, $$ = 600 - 450 = 150 $$