Number System Aptitude Important Formulas, Definitions, & Examples:
Overview:
| Topic Included: | Formulas, Definitions & Exmaples. |
| Main Topic: | Quantitative Aptitude. |
| Quantitative Aptitude Sub-topic: | Number System Aptitude Notes & Questions. |
| Questions for practice: | 10 Questions & Answers with Solutions. |
This chapter of the number system is the most important chapter in the Quantitative Aptitude section. The students are advised to prepare each topic of this chapter, topics of this chapter will help you to solve the questions of other chapters of aptitude too.
What is a Number:
A number is an arithmetic value expressed by a group of digits to represent a particular quantity and used for counting, measuring, and calculation. A group of digits is called numeral, and we have 10 digits to use for counting, and they are 0, 1, 2, 3, 4, 5, 6, 7, 8, and 9.
Concept of Number Line:
The number line is a straight line that starts from point zero, and goes to infinity on the right side, and goes to negative infinity on the left side as given below.
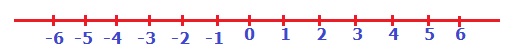
We can find the distance between the two numbers by subtracting the lower number from the higher number. For example the distance between the numbers 4 and -3 will be = 4 - (-3) = 7.
What is the Number System:
The number system is a way to represent the numbers for example, natural numbers, whole numbers, odd numbers, even numbers, rational numbers, irrational numbers, etc.
Types of Numbers:
Here are the types of numbers.
1. Natural Numbers:
The numbers generally used to count something are known as Natural Numbers. It includes numbers from 1 to 9. Zero (0) is not a natural number.
Example: {1, 2, 3, 4, 5, 6, 7, 8, 9}.
2. Whole Numbers:
The natural numbers including zero (0) are known as Whole Numbers. Point to be noted that zero is a whole number but not a natural number.
Example: {0, 1, 2, 3, 4, 5, 6, 7, 8, 9}
3. Even Numbers:
All the numbers which are divisible by 2 are the Even Numbers.
Example: {2, 4, 6, 8, 10, 12, 14.........}
4. Odd Numbers:
All the numbers which are not divisible by 2 are the Odd Numbers.
Example: {1, 3, 5, 7, 9, 11, 13,.........}
5. Integer Numbers:
A set of natural numbers with their negative numbers including zero (0) is known as the set of Integers and each number that belongs to this set is called an integer.
Example: Here is the Set of integers {.........-5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5,.........}
We can further divide these integers into three sets.
A set of positive integers {1, 2, 3, 4, 5,.........}
A set of negative integers {-1, -2, -3, -4, -5,.........}
A set of Non-negative integers {0, 1, 2, 3, 4, 5,.........}
In the above example of integers, left side numbers that are negative are known as Negative Integers, and right-side numbers that are positive are known as positive Integers. One important point is that the number Zero (0) which is neither a positive integer nor a negative integer.
6. Prime Numbers:
The number which is divisible by 1 and itself is known as Prime Number, and also a prime number must have exactly two factors. The number 2 is the only even prime number, and all other prime numbers are odd. The number 2 is also the smallest prime number as 1 is not a prime number.
Example: {2, 3, 5, 7, 11, 13, 17.........}
Why 1 is not a prime number?
1 is not a prime number because any number is a prime number that must have exactly two factors and should be divisible by 1 and itself. The number 1 is divisible by 1 and itself, but the number 1 has only one factor and that is not fulfilling the criteria to be a prime number. This is the reason the number 2 is the smallest prime number.
7. Composite Numbers:
All-natural numbers that are greater than 1 but not the prime numbers are known as composite numbers. A composite number also must have more than two factors.
Example: {4, 6, 8, 9, 10, 12, 14, 15,.........}
Why 1 is not a composite number?
Same reason as we discussed in prime numbers. The number 1 has only one factor, whereas a composite number must have more than two factors.
8. Rational Numbers:
Any number which is in the form of a fraction \((\frac{a}{b})\) of two integers is called the rational number, where denominator "b" must not be zero, and point to be noted that every integer is a rational number too. Example: {\(\frac{1}{2}, \frac{2}{3}, \frac{5}{8}, \frac{6}{9}, -1, -2.........\)}
9. Irrational Numbers:
Any number in the form of decimal and which can not be written in the form of a fraction (a/b) for any integer is called an irrational number.
Example: {\(\pi\), \(\sqrt 2\), \(e\), \(\phi\),.........}
10. Real Numbers:
The combination of rational and irrational numbers are called real numbers. In other words, all the numbers which can be represented on the number line are called real numbers.
Example: {\(\frac{1}{2}, \pi, \frac{2}{3}, \sqrt 2, \frac{5}{8}, \frac{6}{9}.........\)}
Some Important Points:
Even number + Even number = Even number
Even number - Even number = Even number
Odd number + Odd number = Even number
Odd number - Odd number = Even number
Even number + Odd number = Odd number
Even number - Odd number = Odd number
Odd number + Even number = Odd number
Odd number - Even number = Odd number
Even number \(\times\) Even number = Even number
Odd number \(\times\) Odd number = Odd number
Even number \(\times\) Odd number = Even number